Pyramid
Definition
A pyramid is a polyhedron composed of a polygon \(A_1A_2…A_n\) and \(n\) triangles with a common vertex \(P\) (not lying in the plane of the polygon) and sides opposite it, coinciding with the sides of the polygon. Designation: \(PA_1A_2…A_n\). Example: pentagonal pyramid \(PA_1A_2A_3A_4A_5\).
Triangles \(PA_1A_2, \PA_2A_3\), etc. are called the lateral faces of the pyramid, segments \(PA_1, PA_2\), etc. – side edges, polygon \(A_1A_2A_3A_4A_5\) – base, point \(P\) – vertex.
The height of the pyramid is the perpendicular descended from the top of the pyramid to the plane of the base.
A pyramid with a triangle at its base is called a tetrahedron.
A pyramid is called regular if it has a regular polygon at its base and one of the following conditions is met:
\((a)\) the lateral edges of the pyramid are equal;
\((b)\) the height of the pyramid passes through the center of the circle circumscribed near the base;
\((c)\) the side ribs are inclined to the plane of the base at the same angle.
\((d)\) the side faces are inclined to the plane of the base at the same angle.
A regular tetrahedron is a triangular pyramid, all of whose faces are equal equilateral triangles.
Theorem
Conditions \((a), (b), (c), (d)\) are equivalent.
Proof
Let's find the height of the pyramid \(PH\). Let \(\alpha\) be the plane of the base of the pyramid.
1) Let us prove that \((a)\) implies \((b)\). Let \(PA_1=PA_2=PA_3=…=PA_n\).
Because \(PH\perp \alpha\), then \(PH\) is perpendicular to any line lying in this plane, which means that the triangles \(PA_1H, PA_2H, PA_3H,..., PA_nH\) are right-angled. This means that these triangles are equal in common leg \(PH\) and hypotenuse \(PA_1=PA_2=PA_3=...=PA_n\). So, \(A_1H=A_2H=…=A_nH\). This means that the points \(A_1, A_2, …, A_n\) are at the same distance from the point \(H\), therefore, they lie on the same circle with the radius \(A_1H\). This circle, by definition, is circumscribed about the polygon \(A_1A_2…A_n\).
2) Let us prove that \((b)\) implies \((c)\).
Similar to the first point, the triangles \(PA_1H, PA_2H, PA_3H,…, PA_nH\) are right-angled and equal on two sides. This means that their angles are also equal, therefore, \(\angle PA_1H=\angle PA_2H=...=\angle PA_nH\).
3) Let us prove that \((c)\) implies \((a)\).
Similar to the first point, the triangles \(PA_1H, PA_2H, PA_3H,…, PA_nH\) are rectangular both along the leg and the acute angle. This means that their hypotenuses are also equal, that is, \(PA_1=PA_2=PA_3=...=PA_n\).
4) Let us prove that \((b)\) implies \((d)\).
Because in a regular polygon the centers of the circumscribed and inscribed circles coincide (generally speaking, this point is called the center of a regular polygon), then \(H\) is the center of the inscribed circle. Let's draw perpendiculars from the point \(H\) to the sides of the base: \(HK_1, HK_2\), etc. These are the radii of the inscribed circle (by definition). Then, according to TTP (\(PH\) is a perpendicular to the plane, \(HK_1, HK_2\), etc. are projections perpendicular to the sides) inclined \(PK_1, PK_2\), etc. perpendicular to the sides \(A_1A_2, A_2A_3\), etc. respectively. This means that, by definition, \(\angle PK_1H, \angle PK_2H\) are equal to the angles between the side faces and the base. Because triangles \(PK_1H, PK_2H, …\) are equal (as rectangular on two sides), then the angles \(\angle PK_1H, \angle PK_2H, …\) are equal.
5) Let us prove that \((d)\) implies \((b)\).
Similar to the fourth point, the triangles \(PK_1H, PK_2H, …\) are equal (as rectangular along the leg and acute angle), which means the segments \(HK_1=HK_2=…=HK_n\) are equal. This means, by definition, \(H\) is the center of a circle inscribed in the base. But because For regular polygons, the centers of the inscribed and circumscribed circles coincide, then \(H\) is the center of the circumscribed circle. Chtd.
Consequence
The lateral faces of a regular pyramid are equal isosceles triangles.
Definition
The height of the lateral face of a regular pyramid, drawn from its vertex, is called the apothem. The apothems of all lateral faces of a regular pyramid are equal to each other and are also medians and bisectors.
Important Notes
1. The height of a regular triangular pyramid falls at the point of intersection of the heights (or bisectors, or medians) of the base (the base is a regular triangle).
2. The height of a regular quadrangular pyramid falls at the point of intersection of the diagonals of the base (the base is a square).
3. The height of a regular hexagonal pyramid falls at the point of intersection of the diagonals of the base (the base is a regular hexagon).
4. The height of the pyramid is perpendicular to any straight line lying at the base.
Definition
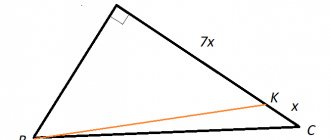
A pyramid is called rectangular if one of its side edges is perpendicular to the plane of the base.
Important Notes
1. In a rectangular pyramid, the edge perpendicular to the base is the height of the pyramid. That is, \(SR\) is the height.
2. Because \(SR\) is perpendicular to any straight line from the base, then \(\triangle SRM, \triangle SRP\) are right triangles.
3. Triangles \(\triangle SRN, \triangle SRK\) are also rectangular. That is, any triangle formed by this edge and the diagonal emerging from the vertex of this edge lying at the base will be rectangular.
\[{\Large{\text{Volume and surface area of the pyramid}}}\]
Theorem
The volume of the pyramid is equal to a third of the product of the area of the base and the height of the pyramid: \[V_{\text{pyramid}}=\dfrac13 S_{\text{base}}\cdot h\]
Consequences
Let \(a\) be the side of the base, \(h\) be the height of the pyramid.
1. The volume of a regular triangular pyramid is equal to \(V_{\text{right.triangular.pir.}}=\dfrac{\sqrt3}{12}a^2h\),
2. The volume of a regular quadrangular pyramid is equal to \(V_{\text{right.four.py.}}=\dfrac13a^2h\).
3. The volume of a regular hexagonal pyramid is equal to \(V_{\text{right hex.pyra.}}=\dfrac{\sqrt3}{2}a^2h\).
4. The volume of a regular tetrahedron is equal to \(V_{\text{right tetrahedron}}=\dfrac{\sqrt3}{12}a^3\).
Theorem
The area of the lateral surface of a regular pyramid is equal to the half product of the perimeter of the base and the apothem.
\[{\Large{\text{Frustum}}}\]
Definition
Consider an arbitrary pyramid \(PA_1A_2A_3…A_n\). Let us draw a plane parallel to the base of the pyramid through a certain point lying on the side edge of the pyramid. This plane will split the pyramid into two polyhedra, one of which is a pyramid (\(PB_1B_2...B_n\)), and the other is called a truncated pyramid (\(A_1A_2...A_nB_1B_2...B_n\)).
The truncated pyramid has two bases - polygons \(A_1A_2...A_n\) and \(B_1B_2...B_n\), which are similar to each other.
The height of a truncated pyramid is a perpendicular drawn from some point of the upper base to the plane of the lower base.
Important Notes
1. All lateral faces of a truncated pyramid are trapezoids.
2. The segment connecting the centers of the bases of a regular truncated pyramid (that is, a pyramid obtained by cross-section of a regular pyramid) is the height.