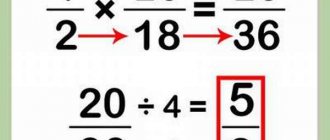Division with remainder and partial quotient
But it is not always possible to divide one number by another. Or rather, it is not always possible to do this completely. For example, 37 cannot be divided by 5, because there is no such natural number that if multiplied by 5, we would get 37. In this case, they say that 37 is not divisible by 5.
For example, if we want to distribute all 37 apples equally among five children, then we will not be able to do this. We will be able to distribute (use from the total number of apples) only 7 apples to each ( \(\textcolor{red} {7\cdot 5=35}\) ), and we will have 2 apples left ( \(\textcolor{red} { 37-35=2}\) ).
In this case, the action of division also consists of the dividend (in our case 37) and the divisor (5). The resulting number 7 is called an incomplete quotient , because we were not able to divide the entire divisible number into the required number of parts. And the difference between the total dividend (37) and the units used from it (35), that is, the number 2, is called the remainder.
So, division with a remainder is finding the largest integer, multiplying it by the divisor, we get a number that is as close as possible to the dividend, but does not exceed it. This required number is called an incomplete quotient . The difference between the dividend and the partial quotient is called the remainder .
The remainder is always less than the divisor!
From this follows the general form of the action of dividing natural numbers for the cases of division without a remainder and with a remainder . Dividing an integer a (dividend) by an integer b (divisor) means finding numbers c and d for which the following relations hold: \(\textcolor{red} {a=b\cdot c+d}\) ; \(\textcolor{red} {d . If \(\textcolor{red} {d=0}\) then a be divisible by b without remainder.
Components of the action of division with a remainder:
Problems that can be solved using division
In high school mathematics courses, division is most often used when solving problems such as when you need to:
- Find out how many times one number is less and more than another? This question may sound different: how many times is the smaller number contained (placed) in the larger one? Or: how many times can a smaller number fit into a larger number? For example: how many five-gram sugar sticks are in a kilogram package? (1000 g: 5 g = 200 pcs.).
- Divide the number into a given number of equal parts. For example: how many grams of sugar will be in each bag if you pour a kilogram of sugar equally into 5 identical bags? (1000 g: 5 pieces = 200 g).
- Decrease a number by a specified number of times. For example: to prepare a dish for 5 people, 1 kg of sugar was used, but how much sugar would be needed to prepare the same dish for one person? (1000 g: 5 people = 200 g).
Relationship between division and multiplication, addition and subtraction
When we find the product of two numbers, these numbers are known to us, and we are required to find the result of the multiplication action. When dividing (without a remainder), we know the product of two numbers, but we need to find a number that, when multiplied by a known given number, gives this same product.
Therefore, the action of division is the inverse of the action of multiplication.
The converse is also true: the action of multiplication is the opposite of the action of division. Thus:
Multiplication and division are mutually inverse operations.
The connection between division and multiplication, as well as addition and subtraction, is clearly visible if we consider how division can be performed using these actions.
Let's look at them using an example: 345 divided by 69.
Dividing two numbers using addition
To find out using addition how many times the number 69 is contained in 345, you need to add 69 sequentially until we get the number we need:
\(\textcolor{red} {69+69=138}\) ; \(\textcolor{red} {138+69=207}\); \(\textcolor{red} {207+69=276}\); \(\textcolor{red} {276+69=345}\).
The number 69 was added only 5 times, which means \(\textcolor{red} {345\div 69=5}\) .
Dividing two numbers using subtraction
Similar to the previous method, we can find out how many times the number 345 contains the number 69 by subtraction. To do this, we will sequentially subtract the number 69 from 345 until we get zero, and count the number of actions:
\(\textcolor{red} {345-69=276}\); \(\textcolor{red} {276-69=207}\); \(\textcolor{red} {207-69=138}\); \(\textcolor{red} {138-69=69}\); \(\textcolor{red} {69-69=0}\).
That is, 69 can be subtracted from 345 5 times, so \(\textcolor{red} {349\div 69=5}\).
Dividing two numbers using multiplication
Using multiplication, you can find out the answer to our question by sorting through the factor of the number 69 until we get the given 345:
\(\textcolor{red} {69\cdot 2=138}\); \(\textcolor{red} {69\cdot 3=207}\); \(\textcolor{red} {69\cdot 4=276}\); \(\textcolor{red} {69\cdot 5=345}\).
The required quotient is equal to the resulting multiplier of the number 69, that is, 5.
But these three methods are very cumbersome, especially if the quotient is a very large number. You need to know them only in order to understand the essence of the action of division, the essence of the problems that are solved through it.
Mathematics lesson “Multiplication and division of natural numbers” 5th grade
Goals:
Educational:
- Summarize and systematize material on this topic;
- To teach how to generalize knowledge and draw conclusions based on compulsory level material;
- Carry out diagnostics of the assimilation of the system of knowledge and skills and its application to perform practical tasks at a standard level with a transition to a higher level;
Educational:
- Promote rational organization of labor;
Educational:
- Develop cognitive processes, memory, imagination, thinking, attention, observation, intelligence;
- Develop self-esteem in choosing a path;
- To increase students' interest in non-standard tasks and to form a positive motive for learning.
Topic content: this topic of the mathematics program of any current textbook from the Federal set.
Lesson type: lesson of generalization and systematization.
Lesson structure:
- motivational conversation followed by goal setting;
- updating basic knowledge;
- practical tasks;
- summing up the lesson;
1) Teacher: Good afternoon, guys! Today we will conduct a lesson on generalizing knowledge on the topic “Multiplication and division of natural numbers”, which will allow us to identify the level of your knowledge and skills on this topic when performing practical tasks. I hope that today’s lesson will in many ways become a development lesson for you and will increase your interest in the subject of mathematics. So, is everyone ready? Then - let's go!
A) We start by repeating previously studied material:
B) In front of you are sheets of paper with mathematical dictation tasks. You only have to give the answers.
C) Hand in the answer sheets. Now check whether you answered the dictation tasks correctly.
D) Physical exercise.
I will now read out examples with answers to you. If you agree with me, then raise your hands up, and if not, then stretch them forward.
- If you agree with me, then tilt your head forward, if not, then tilt your head back.
E) Each row needs to complete various tasks to apply the rule about the order of actions in calculations.
Let's check what answers you received. The student from each row gives an answer and explains his actions when solving examples.
E) Some fun math.
G) Independent work on 2 options.
- Calculate:
- Solve the equations:
- Solve the problem using the equation:
Summarizing:
– You did a great job in class. I hope you will not forget this material; it will be useful to you throughout your training. Everyone probably remembers the saying: “Repetition is the mother of learning!” Mathematics is no exception. And in order to assimilate it well, you must constantly repeat what you have learned.
General principle of column division
If the quotient of two numbers is a multi-digit number, it is found by dividing into a column. It is also called corner division.
Let's solve the example \(\textcolor{red} {295383\div 34}\).
First of all, you need to find out the number of digits in the quotient and the first incomplete dividend; I described in detail how to find them in this article. In our case, the first incomplete dividend is equal to 295 thousand, and in the quotient there will be 4 digits.
Next, we write the known division components as follows:
and start the calculation:
1. We take the first incomplete dividend and try to divide it by the divisor.
This is where the method of finding an unambiguous quotient comes in handy. Using it, we find that in 295 thousand the divisor 34 is contained entirely 8 thousand times.
We write the first digit found in the thousands place into the quotient, and under the incomplete dividend we write the result of the product of the incomplete quotient and the divisor. And we immediately find the remainder of this action, i.e. subtract the result of this product from the incomplete quotient.
As a result of multiplying the first found digit of the quotient by the divisor, we got \(\textcolor{red} {8\cdot 37=272}\). We write it under 295 and find the difference: \(\textcolor{red} {295-272=23}\). This means that 23 thousand remain undivided.
As another self-test, you need to compare the resulting difference with the divisor. If it is less than the divisor, then we are on the right track, but if the difference is equal to or greater than the divisor, then we either incorrectly found the quotient number, or made an error when multiplying by the divisor or when finding the remainder.
2. The remaining undivided 23 thousand represents 230 hundreds. We add to them those 3 hundreds that are contained in the dividend (they say: take away five) and we get the second incomplete dividend 233 hundreds.
We find the result of dividing the second incomplete dividend by the divisor. 233 hundreds divided by 34 is 6 hundreds. This means that in the hundreds place of the quotient there will be the number 6. We multiply it by the divisor 34, we get 204 and another 29 undivided hundreds.
3. 29 undivided hundreds are 290 tens. We add (subtract) 8 tens of the dividend to them, we get the third incomplete dividend of 298 tens.
When dividing the second incomplete dividend of 298 tens by a divisor of 34, we get 8 tens, and another 26 undivided tens (as in the previous steps, I multiplied 8 by 34 and subtracted the result from 298). Therefore, in particular, in the tens place we write the number 8.
4. And finally, 26 tens are 260 prime units. We add (remove) 3 units of the dividend to them and get the fourth incomplete dividend of 263 units.
Dividing 263 units by 34 gives 7 full units and 25 undivided ones. Having written the last digit of the units place in the quotient, we get the final answer of the action \(\textcolor{red} {295383\div 34=8687}\) and 25 as the remainder.
Let's look at another example. \(\textcolor{red} {25326\div 63}\).
The first incomplete dividend will be 253 hundreds, the number of digits in the quotient will be 3.
We divide 253 hundreds by 63, we get 4 full hundreds and an undivided 1 hundred as a remainder.
1 hundred = 10 tens, add (remove) 2 tens from the dividend, we get the second incomplete dividend 12 tens.
But 12 is not completely divisible into 63 parts, that is, there is not a single whole ten in each part. This means that we must write 0 in the tens place, since all 12 tens turned out to be undivided. And to these 12 tens (i.e. 120 hundreds) add (take away) 6 units of the dividend.
So, remember that each incomplete dividend forms one digit of the corresponding digit in the quotient and that even if the incomplete dividend is less than the divisor, then in the quotient you still need to write down the zero result of this action.
Divide 126 units by 63, resulting in 2 units without a remainder. Now we can write down the final division answer \(\textcolor{red} {25326\div 63=402}\).
So, in general, the long division algorithm looks like this: 1. Find the first incomplete dividend and the number of digits in the quotient. 2. Divide the incomplete dividend by the divisor. The number obtained as a result of division is written below the line under the divisor. 3. Multiply the resulting number by the divisor, writing the result under the incomplete dividend. 4. Put a minus sign between them and perform the action. 5. To the resulting difference we add the digit of the next digit (if there is one) and get the second incomplete dividend. 6. We carry out steps 2-5 until there are not a single missing digit left in the dividend. 7. If the incomplete dividend cannot be divided by a divisor, then 0 is put in the quotient and the next digit is added to this incomplete dividend.
5.5.6. Division by decimal
I. To divide a number by a decimal fraction, you need to move the commas in the dividend and divisor as many digits to the right as there are after the decimal point in the divisor, and then divide by the natural number.
Examples .
Perform division: 1) 16,38:0,7; 2) 15,6:0,15; 3) 3,114:4,5; 4) 53,84:0,1.
Solution.
Example 1) 16,38:0,7.
In the divisor of 0.7 there is one digit after the decimal point, so let’s move the commas in the dividend and divisor one digit to the right.
Then we will need to divide 163.8 by 7 .
Let's perform the division according to the rule for dividing a decimal fraction by a natural number.
We divide as natural numbers are divided. As soon as we remove the number 8 - the first digit after the decimal point (i.e. the number in the tenths place), we immediately put a comma in the quotient and continue the division.
Answer: 23.4.
Example 2) 15,6:0,15.
We move the commas in the dividend ( 15.6 ) and divisor ( 0.15 ) two digits to the right, since in the divisor 0.15 there are two digits after the decimal point.
We remember that you can add as many zeros as you like to the decimal fraction on the right, and this will not change the decimal fraction.
Then:
15,6:0,15=1560:15.
We perform division of natural numbers.
Answer: 104.
Example 3) 3,114:4,5.
Let's move the commas in the dividend and divisor one digit to the right and divide 31.14 by 45 according to the rule for dividing a decimal fraction by a natural number.
So:
3,114:4,5=31,14:45.
In the quotient, we put a comma as soon as we remove the number 1 in the tenths place. Then we continue dividing.
To complete the division we had to add a zero to the number 9 - the difference between the numbers 414 and 405 . (we know that zeros can be added to the right side of a decimal fraction)
Answer: 0.692.
Example 4) 53,84:0,1.
Move the commas in the dividend and divisor one digit to the right.
We get: 538,4:1=538,4.
Let's analyze the equality: 53.84:0.1=538.4. Pay attention to the comma in the dividend in this example and the comma in the resulting quotient. We notice that the comma in the dividend is moved 1 digit to the right, as if we were multiplying 53.84 by 10. (See the video “Multiplying a decimal by 10, 100, 1000, etc.”) Hence the rule for dividing a decimal by 0 ,1; 0.01; 0.001 , etc.
II. To divide a decimal by 0.1; 0.01; 0.001, etc., you need to move the decimal point to the right by 1, 2, 3, etc. digits. (Dividing a decimal by 0.1, 0.01, 0.001, etc. is the same as multiplying that decimal by 10, 100, 1000, etc.)
Examples.
Perform division: 1) 617,35:0,1; 2) 0,235:0,01; 3) 2,7845:0,001; 4) 26,397:0,0001.
Solution.
Example 1) 617,35:0,1.
According to Rule II , dividing by 0.1 is equivalent to multiplying by 10 , and move the comma in the dividend one digit to the right :
1) 617,35:0,1=6173,5.
Example 2) 0,235:0,01.
Dividing by 0.01 is equivalent to multiplying by 100 , which means we move the comma in the dividend two digits to the right :
2) 0,235:0,01=23,5.
Example 3) 2,7845:0,001.
Since dividing by 0.001 is equivalent to multiplying by 1000 , we move the decimal point 3 digits to the right :
3) 2,7845:0,001=2784,5.
Example 4) 26,397:0,0001.
Dividing a decimal fraction by 0.0001 is the same as multiplying it by 10000 (move the decimal point 4 digits to the right ). We get:
4) 26,397:0,0001=263970.
Watch the video “Divide by Decimals”
Division by numbers ending in zeros
As with multiplication, dividing numbers is made easier if the divisor ends with one or more zeros. Let's consider two possible cases:
- quotient – when the divisor is one with zeros
- common – when the divisor is any number ending in zeros.
Let's consider the first case.
Division by one with any number of zeros
A unit with any number of zeros is nothing more than a unit of the corresponding digit. For example, 10 is 1 unit in the tens place, 1000 is one unit in the thousands place, 10000000 is 1 unit in the tens place, etc.
Therefore, divide the number, for example, by 10, 1000, 10000000, etc. - this means determining how many tens, thousands, tens of millions it contains. And how to find out how many units of any rank are contained in any number, I already explained in the lesson ranks and classes. To complete the action of division, you only need to write down in the remainder the number that is obtained from the digits we discard.
For example:
\(\textcolor{red} {75427916\div 10=7542791}\) (remainder 6); \(\textcolor{red} {75427916\div 1000=75427}\) (remainder 916); \(\textcolor{red} {75427916\div 10000000=7}\) (remainder 5427916).
Write down: To divide a number by one with any number of zeros , you need to count as many digits in the dividend on the right as there are zeros in the divisor; then all the numbers to the left of the division will form the quotient, and those to the right will be the remainder.
Division by a number ending in zeros
Let's look at the example of \(\textcolor{red} {284556\div 2800}\).
The divisor here is nothing more than 28 hundred. It is logical to assume that these 28 hundreds can be contained at least once only in the hundreds of the dividend. This means that we need to determine how many hundred units there are in the dividend and divide them by 28 units of the hundreds place of the dividend. And the discarded digits of tens and prime units will be added to the remainder.
Among 284556 there are only 2845 hundreds and 56 units. Divide 2845 hundreds by 28 hundreds, we get the quotient of 101 and 17 hundreds undivided. By adding 56 units from the dividend to the undivided 17 hundreds, we get 1756. In this number, the divisor 2800 does not fit even once, which means that 1756 is the remainder: \(\textcolor{red} {284556\div 2800=101}\) (remainder 1756).
Write down: To divide a number by a number ending in zeros , you need to mentally discard the zeros in the divisor, and mentally discard the same number of digits in the dividend as there are zeros in the divisor. Divide the resulting number in the dividend by the resulting number in the divisor, and to the remainder add (take away) those digits of the dividend that were discarded earlier.
Dividing a fraction by a number.
To divide a fraction by a number, you need to multiply the denominator of the fraction by the number.
\(\bf \frac{a}{b} \div n = \frac{a}{b} \div \frac{n}{1} = \frac{a}{b} \times \frac{1} {n}\\\)
Let's look at an example:
Divide the fraction by the natural number \(\frac{4}{7} \div 3\).
As we already know, any number can be represented as a fraction \(3 = \frac{3}{1}\).
\(\frac{4}{7} \div 3 = \frac{4}{7} \div \frac{3}{1} = \frac{4}{7} \times \frac{1}{3 } = \frac{4 \times 1}{7 \times 3} = \frac{4}{21}\\\)
Checking division
Since the dividend is the divisor multiplied by the quotient and plus the remainder, which follows from the definition of division, the result of division can be checked by multiplication.
For example:
After we multiplied the quotient 241 by the divisor 33, and added the remainder 9 to the resulting product, we received the number 7962, which is equal to the dividend. This means that we can say with great confidence that the division operation was performed correctly.
If the division action does not result in a remainder, then the division can be checked by division. Indeed, if the dividend is the product of a divisor and a quotient, then by dividing the dividend by the quotient (one of the factors), we must obtain the second factor, that is, the divisor.
For example:
Linear equations for grade 5
One of the most important skills when entering 5th grade
is the ability to solve simple equations.
Since 5th grade is not yet so far from elementary school, there are not so many types of equations that a student can solve. We will introduce you to all the main types of equations that you need to be able to solve if you want to enter a physics and mathematics school
.
Type 1: “onion” These are equations that you are almost likely to encounter when entering any school
or a 5th grade club as a separate task. They are easy to distinguish from others: in them the variable is present only once. For example, or. They are solved very simply: you just need to “get” to the unknown, gradually “removing” everything unnecessary that surrounds it - as if peeling an onion - hence the name. To solve it, just remember a few rules from the second class. Let's list them all:
Addition
- term1 + term2 = sum
- term1 = sum - term2
- term2 = sum - term1
Subtraction
- minuend - subtrahend = difference
- minuend = subtrahend + difference
- subtrahend = minuend - difference
Multiplication
- factor1 * factor2 = product
- factor1 = product : factor2
- factor2 = product : factor1
Division
- dividend : divisor = quotient
- dividend = divisor * quotient
- divisor = dividend : quotient
Let's look at an example of how to apply these rules. Note that we divide by and get . In this situation, we know the divisor and the quotient. To find the dividend, you need to multiply the divisor by the quotient: We have become a little closer to the quotient itself. Now we see that we add to and get . This means that in order to find one of the terms, you need to subtract the known term from the sum: And another “layer” is removed from the unknown! Now we see a situation with a known value of the product () and one known multiplier (). Now the situation is “minuend - subtrahend = difference” And the last step is the known product () and one of the factors ()
Type 2: equations with brackets Equations of this type are most often found in problems - 90% of all problems for admission to grade 5
.
Unlike “onion equations,”
a variable here can appear several times, so it is impossible to solve it using the methods from the previous paragraph.
Typical equations: or The main difficulty is opening the brackets correctly. After we have managed to do this correctly, we should reduce similar terms (numbers to numbers, variables to variables), and after that we get the simplest “onion equation”
that we can solve. But first things first.
Expanding parentheses
. We will give several rules that should be used in this case. But, as practice shows, the student begins to open the brackets correctly only after 70-80 completed problems. The basic rule is this: any factor outside the brackets must be multiplied by each term inside the brackets. And the minus sign in front of the bracket changes the sign of all the expressions inside. So, the basic rules of disclosure:
Bringing similar
.
Here everything is much easier: you need to, by transferring the terms through the equal sign, ensure that on one side there are only terms with the unknown, and on the other - only numbers. The basic rule is this: each term transferred through changes its sign - if it was with, it will become with, and vice versa. After a successful transfer, it is necessary to count the total number of unknowns, the total number on the other side of the equality than the variables, and solve a simple “onion equation”
.
Let's give an example: (let's open the parentheses. Pay attention to the change of signs!) (let's perform multiplications) (transfer , and through the equal sign - they will “turn” into , and ) (calculate the total number on the right and the number on the left) (situation “known factor and product ")
Having mastered these two types of equations, you can be sure that you will be able to solve a good half of all tasks in the introductory Olympiad for 5th grade
.
Properties of division
I will present the properties of division in two groups:
- actions with one and zero;
- distribution properties of division.
Let's look at each group in more detail.
Operations of division with one and zero
When a number is divided by one, the same number is obtained.
Indeed, dividing a number by one means finding out how many units are contained in a given number. And the number of units in a number is nothing more than the number itself.
And, for example, if 10 apples need to be distributed to one person (10 divided by 1), then he will get all 10 apples, right?
When dividing like numbers (numbers by an equal number), the result will be 1 (one).
In fact, if all the units of a certain number are divided into a number of parts equal to the number of units of this number, then each part will receive 1 unit.
For example, if 20 apples are distributed to 20 schoolchildren, then each will receive 1 apple.
When zero is divided by any number other than zero, the result is zero.
Dividing zero by a number means finding a number that, when multiplied by a given divisor, will result in zero. And there is only one such number - zero.
You cannot divide by zero, that is, zero cannot act as a divisor.
When dividing any numbers, the divisor can be any number except zero.
Let's consider two cases: when only the divisor is zero, and when the dividend and the divisor are both zeros.
Let the dividend be equal to any number other than zero, for example, 12. Dividing the number 12 by zero means finding a number that, when multiplied by 0, would result in the number 12. But as you know, if any number is multiplied by 0, then we also get zero. Therefore, the number we need does not exist.
Let's assume that the dividend and the divisor are both zeros. In this case, we need to find a number that, when multiplied by 0, would result in 0. And since no matter what number we take, when multiplied by 0 , we also get zero, then any number from an infinite set of numbers can be quotient, therefore, there cannot be any definite result from such a division.
Distributive properties of division
To find the quotient of a sum divided by a number , you need to divide each term by this number and find the sum of the resulting quotients. \(\textcolor{red} {(a+b+c)\div d=a\div d+b\div d+c\div d}\). This implies that all division operations are obtained without a remainder.
For example, to find the result of dividing the sum \(\textcolor{red} {24+16+48}\) by 8, that is, to determine how many eights are in the sum of these numbers, we find out how many times the eight is contained separately in each from numbers, and then add up the results.
So, in 24 there are 3 eights, in 16 - two, in 48 - six, total \(\textcolor{red} {3+2+6=11}\). And if we first find the value of the entire sum \(\textcolor{red} {24+16+48=88}\), and divide it by 8, then the answer will also be \(\textcolor{red} {88\div 8= eleven}\).
To find the quotient of dividing the difference by a number , you need to divide by this number separately, first the minuend, and then the subtrahend, and then find the difference between the first quotient and the second. \(\textcolor{red} {(ab)\div c=a\div cb\div c}\) It is also assumed that when dividing the minuend and subtrahend by a number, no remainders are obtained.
For example: \[\textcolor{red} {(36-24)\div 6=36\div 6-24\div 6=6-4=2}\] The number 36 consists of 6 sixes, and 24 - of 4 sixes , and taking 4 sixes from 6 sixes, we get 2 sixes. The same result will be if we first subtract 24 units from 36 (12 will remain), and then find how many sixes this difference contains: \(\textcolor{red} {12\div 6=2}\).
To find the quotient of dividing a product by a number , you need to divide only one of the factors by it, and multiply the result by the remaining unchanged. \(\textcolor{red} {(a\cdot b\cdot c)\div d=a\div d\cdot b\cdot c=b\div d\cdot a\cdot c=c\div d\cdot a \cdot b}\).
In fact, dividing, for example, \(\textcolor{red} {20\cdot 25\cdot 35}\) by 5 means reducing the product by 5 times. And since if we reduce one of the factors by a certain number of times, then the product will also decrease by the same number of times, then we just need to divide any of the numbers 20, 25 or 35 by 5 to get the answer: \(\textcolor{red} { (20\cdot 25\cdot 35)\div 5=20\div 5\cdot 25\cdot 35=3500}\).
To find the quotient of a number divided by a product , you need to divide this number by the first factor, divide the result of division by the second factor, divide the resulting quotient by the third, and so on. \(\textcolor{red} {a\div (b\cdot c\cdot d\cdot e)=a\div b\div c\div e}\). It is assumed that with all these divisions no remainders are obtained.
Let's say you need to divide 30 by the product \(\textcolor{red} {2\cdot 3}\). We know that division is the decomposition of a number into equal parts. This means that dividing 30 units by 2, we find that each of the 2 equal parts contains 15 units. After this, we divide these 15 units into 3 equal parts, and find out that each of them contains 5 units.
The figure clearly shows that in the end, after applying this rule, the number 30 turned out to be divided into 6 equal parts.
What fractions cannot be divided?
This is a very interesting question. Only existing fractions can be divided. What does it mean? There are a number of fractions that are not considered in 5th grade mathematics and school mathematics in general - these are fractions with a denominator equal to 0. Such fractions do not exist, and therefore cannot be divided by them.
Just like you can't divide by zero. That is, if the divisor is a fraction with a numerator equal to zero, then it will not be possible to divide by such a divisor.
If you flip a fraction with a numerator equal to zero, then instead of a number equal to zero, you get a fraction with a denominator of zero. Such a number does not exist, and therefore cannot be divided by it. Therefore, it does not matter which division method you choose: you still cannot divide by such numbers.
Conversely, if the dividend is equal to zero or a fraction with a numerator equal to zero, then you can safely divide the fraction by the fraction and get zero as a result.