"Coordinate plane" 6th grade. presentation for an algebra lesson (6th grade) on the topic
Slide 1
Teacher MBOU Secondary School No. 61, Voronezh Aksenova N.V. Lesson topic: “Coordinate plane” Mathematics 6th grade
Slide 2
To correctly take your place in the cinema, you need to know two coordinates - row and seat Cinema 1st row 2nd row 3rd row 4th row 5th row 6 5 4 3 2 1 7 8 9 10 11 12 6 5 4 3 2 1 7 8 9 10 11 12 6 5 4 3 2 1 7 8 9 10 11 12 6 5 4 3 2 1 7 8 9 10 11 12 6 5 4 3 2 1 7 8 9 10 11 12 SCREEN 3rd row 10th place
Slide 3
A B C D E F G H I K 10 9 8 7 6 5 4 3 2 1 D 5
Slide 4
Chess f6 d5
Slide 5
Using a coordinate grid, pilots and sailors determine the location of objects.
Slide 6
Rene Descartes is a French philosopher, mathematician, physicist and physiologist. (1596-1650). Author of the coordinate plane, which is why it is often called the Cartesian coordinate system.
Slide 7
0 1 2 3 4 5 -5 -4 -3 -2 -1 x-axis ordinate axis -1 -2 -3 -4 1 2 3 4 Y RECTANGULAR (CARTESIAN) COORDINATE SYSTEM O X origin
Slide 8
0 1 2 3 4 5 -5 -4 -3 -2 -1 -1 -2 -3 -4 1 2 3 4 YX Determination of the coordinates of point A (4;2) B (-3; - 1) abscissa ordinate X= 4 U=2
Slide 9
0 1 2 3 4 5 -5 -4 -3 -2 -1 -1 -2 -3 -4 1 2 3 4 YX Determine the coordinates of points A B C D E F (-4;3) (2;2) ( 4;-1) (-5;0) (-3 ;-2) (0;-2)
Slide 10
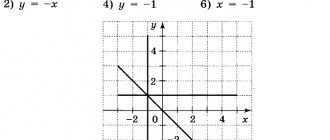
Construct a figure by sequentially connecting points 1) (- 8; 1), (- 6; 2), (- 2; 0), (1; 2), (5; 1), (7; - 4), (9 ; - 3). 2) (- 2; 6), (0; 8), (3; 7), (5; 5), (7; 7). 3) (1; 2), (3; 9), (4; 8), (5; 8), (6; 9), (6; 10), (5; 11), (4; 11), (3; 10), (3; 9). 0 YX 2 4 6 8 10 12 14 -2 -4 -6 -8 -10 -12 2 8 12 4 6 10 -2 -4 -6 -8 -10 -12 -14
Slide 11
Construct figures at given points Mouse 1) (3; - 4), (3; - 1), (2; 3), (2; 5), (3; 6), (3; 8), (2; 9 ), (1; 9), (- 1; 7), (- 1; 6), (- 4; 4), (- 2; 3), (- 1; 3), (- 1; 1), (- 2; 1), (-2; - 1), (- 1; 0), (- 1; - 4), (- 2; - 4), (- 2; - 6), (- 3; - 6), (- 3; - 7), (- 1; - 7), (- 1; - 5), (1; - 5), (1; - 6), (3; - 6), ( 3; - 7), (4; - 7), (4; - 5), (2; - 5), (3; - 4). 2) Tail: (3; - 3), (5; - 3), (5; 3). 3) Eye: (- 1; 5). Fox 1) (- 3; 0), (- 2; 1), (3; 1), (3; 2), (5; 5), (5; 3), (6; 2), (7; 2), (7; 1.5), (5; 0), (4; 0), (4; - 1.5), (3; - 1), (3; - 1.5), (4 ; - 2.5), (4.5; - 2.5), (- 4.5; - 3), (3.5; - 3), (2; - 1.5), (2; - 1), (- 2; - 2), (- 2; - 2.5), (- 1; - 2.5), (- 1; - 3), (- 3; - 3), (- 3 ; - 2), (- 2; - 1), (- 3; - 1), (- 4; - 2), (- 7; - 2), (- 8; - 1), (- 7; 0 ), (- thirty). 2) Eye: (5; 2).
Slide 12
0 YX 2 4 6 8 10 12 14 -2 -4 -6 -8 -10 -12 2 8 12 4 6 10 -2 -4 -6 -8 -10 -12 -14
Slide 13
0 YX 2 4 6 8 10 12 14 -2 -4 -6 -8 -10 -12 2 8 12 4 6 10 -2 -4 -6 -8 -10 -12 -14
Slide 14
Swallow (-5; 4), (-7; 4), (-9; 6), (-11; 6), (-12; 5), (-14; 5), (-12; 4), (-14; 3), (-12; 3), (-11; 2), (-10; 2), (-9; 1), (-9; 0), (-8; -2), (0; -3), (3; -2), (19; -2), (4; 0), (19; 4), (4; 2), (2; 3), (6; 9) , (10; 11), (3; 11), (1; 10), (-5; 4), eyes (-10.5; 4.5). Duck (3; 0), (1; 2), (-1; 2), (3; 5), (1; 8), (-3; 7), (-5; 8), (-3; 4), (-6; 3), (-3; 3), (-5; 2), (-5; -2), (-2; -3), (-4; -4), (1 ; -4), (3; -3), (6; 1), (3; 0) and (-1; 5). Elephant 1 (-1; 4), (-2; 1), (-3; 2), (-4; 2), (-4; 3), (-6; 4), (-6; 6) , (-8; 9), (-7; 10), (-6; 10), (-6; 11), (-5; 10), (-4; 10), (-3; 9), (-1; 9.5), (1; 9), (3; 10), (4; 11), (4; 16), (3; 18), (5; 17), (6; 17) , (5; 16), (6; 12), (6; 9), (4; 7), (1; 6), (2; 5), (5; 4), (5; 3), ( 4; 4), (1; 2), (1; 0), (3; -4), (4; -5), (1;-7), (1; -6), (0; -4 ), (-2; -7), (-1.5; -8), (-5; -7), (-4; -6), (-5; -4), (-7;-5 ), (-7; -7), (-6.5; -8), (-10.5; -8), (-10; -7), (-10; -6), (-11; -7), (-11; -8), (-14; -6), (-13; -5), (-12; -3), (-13; -2), (-14; -3 ), (-12; 1), (-10; 3), (-8; 3), (-6; 4), eye (-1; 7). Camel (-10; -2), (-11; -3), (-10.5; -5), (-11; -7), (-12; -10), (-11; -13) , (-13; -13), (-13.5; -7.5), (-13; -7), (-12.5; -5), (-13; -3), (-14 ; -1), (-14; 4), (-15; -6), (-15; -3), (-14; 2), (-11; 4), (-10; 8), ( -8; 9), (-6; 8), (-5; 5), (-3; 8), (-1; 9), (0; 8), (0.5; 6), (0 ,5;4), (3;2,5), (4;3), (5;4), (6;6), (8;7), (9.5;7), (10;6 ), (11.5;5.5), (12;5), (12;4.5), (11;5), (12;4), (11;4), (10;3.5 ), (10.5;1.5), (10;0), (6;-3), (2;-5), (1.5;-7), (1.5;-11), (2.5;-13), (1;-13), (0;-5), (-0.5;-11), (0;-13), (-1.5;-13), (-1.5;-7), (-2;-5), (-3;-4), (-5;-4.5), (-7;4.5), (-9;- 5), (-10;-6), (-9;-12), (-8.5;-13), (-10.5;-13), (-10;-9.5), ( -11;-7), eye (8.5;5.5)
Mathematics lesson “Coordinates. Rectangular coordinate system"; 6th grade
Topic: “Coordinates. Rectangular coordinate system."
Lesson type : Lesson on introducing new material.
Lesson format: Mixed lesson (lecture + practical independent work.
Educational and methodological support: Mathematics 5-9- M. Education, 2011 Bunimovich E.A. Mathematics. Arithmetic. Geometry. 6th grade: textbook for general education institutions. M. Education, 2013
Implementation time: 45 min.
Computer media products. A visual presentation of educational material made in Power Point.
Lesson objectives:
-familiarize yourself with the rectangular coordinate system on a plane
— teach how to freely navigate the coordinate plane;
— build points according to their given coordinates;
— determine the coordinates of a point marked on the coordinate plane;
- perceive coordinates well by ear;
— clearly and accurately carry out geometric constructions;
— development of creative abilities;
- nurturing interest in the subject.
Lesson objectives:
Educational – mastering the algorithm for constructing points on a coordinate line; demonstration of the close connection of subjects (mathematics and computer science).
Developmental – broadening the horizons, improving the information culture of students; development of students' cognitive skills; developing the ability to independently construct one’s knowledge; development of students' creative abilities; development of skills to compare, analyze, generalize, draw conclusions; create a research culture; develop interest in knowledge; transfer theoretical knowledge to practice.
Educational – fostering a sense of responsibility, mutual understanding, mutual support, self-confidence, developing the ability to work in groups.
Necessary equipment and materials for the lesson: computer, projector, screen, blackboard, chalk, cards for practical assignments.
Lesson map
| Commentary on the progress of the lesson. | Time (min). | Slide no. | Development of personal qualities and mental processes. | |
| Reproductive forms of activity. | Productive forms of activity. | |||
| I. Organizing time Greetings. Activation of attention, announcement of the topic of the lesson. | 2 | 1 | Attention, organization. | Curiosity. |
| II. Updating basic knowledge: Frontal survey on the topics “Coordinates”, “Coordinate line”, “Coordinate of a point on a coordinate line” Checking homework for some students. | 4 | 2 | Attention. Memory. | Reasoning, activity, speech accuracy. |
| III. Introduction to the topic of the lesson. Examples of different coordinates. | 3 | 3-8 | Attention. | Reasoning, activity, speech accuracy. |
| IV. Stage of learning new material Creating a problematic situation: each point on a straight line is assigned a number, but we cannot assign a number to each point on a plane. What is the difference between a plane and a straight line? What needs to be done to determine the position of a point on a plane? Hypothesis: for each point on the plane we must associate two numbers that will uniquely identify a specific point. Hypothesis testing . We determine the coordinates of a specific point. We construct a point using the given coordinates. Compilation of notes. | 8 | 9-15 | Attention. | Curiosity. Reasoning. Reasoning. |
| V. Teamwork between teacher and students Solving problems on the topic: “Coordinate plane.” | 10 | 16,17 | Attention. Memory. | Research, listening skills. reasoning, observation. |
| VI . Primary consolidation of the material being studied. Independent work using cards | 10 | 18 | Memory, Attention. | Study. Analyticity. Ability to apply acquired knowledge. |
| VII . Lesson summary. The teacher invites students to summarize the knowledge gained in the lesson, to summarize where this knowledge can be applied. The students summarize. | 3 | 19 | Attention. Memory. | Reasoning, listening skills. reasoning, Clarity of speech. |
| VIII . Presentation and recording of homework by children in the diary. | 3 | 20 | Attention. | |
| IX. Lesson reflection. The teacher invites students to determine the value of the lesson and evaluate their work using emoticons. | 2 | 21 | Attention, memory. | Analyticity. |
| Parting | 22 | |||
Lesson script
I. _ Organizing time
Greetings. Establishing personal contact, joint acceptance and inclusion of a motive for cooperation in the lesson through active communication with students. Preparing the workplace and notebooks for the lesson. Communicating the goals and objectives of the lesson.
II . Updating of reference knowledge
Frontal work. Work orally.
Teacher question: how to turn a straight line into a coordinate line?
On the projector screen is a drawing of a coordinate line with points marked on it.
Working with the drawing, students are asked to answer the following questions:
– What is shown on the board?
– What is a coordinate line?
– Which point is the origin of coordinates?
– What is the coordinate of a point on a line called?
– What numbers are the coordinates of points on a line located:
a) to the right of the origin;
b) to the left of the origin?
– What is the coordinate of the origin?
III . Introduction to the topic of the lesson.
Teacher’s question: “What associations do you have when you say the word “coordinates”?
Children, at the teacher’s prompting, give examples of different coordinates.
Slides showing examples are shown on the screen: the address of a company or person’s residence; contact number; seats in the auditorium; railway ticket; geographical coordinates; chess, checkers; spreadsheet cell address.
IV . The stage of learning new material.
Creating a problematic situation: each point on a straight line is assigned a number, but we cannot assign a number to each point on a plane. What is the difference between a plane and a straight line? What needs to be done to determine the position of a point on a plane?
Hypothesis: for each point on the plane we must associate two numbers that will uniquely identify a specific point.
Hypothesis testing . We determine the coordinates of a specific point. We construct a point using the given coordinates.
Students listen carefully to the teacher’s explanations and write down definitions as the teacher dictates them.
Let's talk again about how the coordinates of a point on the coordinate plane are written.
V. _ Teamwork between teacher and students
Solving problems on the topic: “Coordinate plane.” At this stage, students are expected to work in groups (2-3 people) at the blackboard. The rest listen attentively and, in sync with the guys at the blackboard, complete the assignments in their notebooks.
VI . Primary consolidation of the material being studied. Children do independent work on options using cards.
VII . Lesson summary.
Students answer questions asked by the teacher:
- What are coordinates?
- What lines are called a coordinate system on a plane?
- At what angle do they intersect?
- What point is called the origin of coordinates?
- What plane is called the coordinate plane?
- What is the name of a pair of numbers that determines the position of a point on a plane?
- What is another name for the coordinates of a point on a plane and coordinate lines?
- Tell us how to find the abscissa and ordinate of a point located on a coordinate line.
The teacher notes the activity of the students, thanks them for their work, and announces another lesson on this topic.
VIII . Presentation and recording of homework by children in the diary.
Students write down homework assignments in a diary.
IX . Lesson reflection.
The teacher invites students to determine the value of the lesson, its usefulness.
How do you evaluate your work? Students are encouraged to evaluate their work in class using emoticons. Full text of the material Mathematics lesson “Coordinates.
Rectangular coordinate system"; For grade 6, see the downloadable file . The page contains a fragment.
| Author: Batalshchikova Inga Viktorovna → Kaktus9306 05/21/2016 0 4765 418 | Comment |
Thank you for your mark. If you want your name to be known to the author, log in to the site as a user and click Thank you again. Your name will appear on this page.
Login | Registration
Have an opinion? Leave a comment