Summary of a geometry lesson in 7th grade with presentation. Signs of equality of triangles
Geometry lesson in 7th grade on the topic “Triangles.
Signs of equality of triangles." Abstract with presentation Author: Lyudmila Vasilievna Bitkova, first category mathematics teacher, municipal budgetary educational institution "Forest Basic Secondary School", Lesnoy village of Zubovo - Polyansky district of the Republic of Mordovia. Description of the material: The summary of the presented lesson is a general lesson on the topic “Triangles. Signs of equality of triangles." The purpose of the lesson is to update the material covered and activate it. The lesson allows students to deepen their knowledge of geometry and instills interest in such a serious and at the same time interesting subject. This lesson will be useful for teachers teaching geometry in the 7th grade. The lesson is accompanied by a computer presentation. Lesson topic: “Triangles. Signs of equality of triangles” Lesson objectives: 1) educational: repeat and consolidate students’ knowledge of the formulations of signs of equality of triangles; develop skills: recognize equal triangles, prove their equality, draw conclusions about the equality of some of their elements, practice problem-solving skills using signs of equality of triangles; 2) developing: develop the ability to solve problems using ready-made drawings and with a complete solution, logical thinking and cognitive activity, develop attention, auditory and visual memory, form students’ mathematical speech, as well as self-control and mutual control skills; 3) educational: to cultivate the ability to express one’s point of view, carry out reasoning and proof when solving problems, mathematical culture, the desire to actively participate in work in the lesson, as well as the ability to listen and hear one’s classmates. Lesson objectives: 1. Update basic knowledge on the topic “Triangle”. 2. Check the concept of a triangle. 3. Formulate the criteria for the equality of triangles. 4. Reinforce the material by solving problems using ready-made drawings. 5. Learn to correctly and accurately formulate and solve problems. Lesson type: lesson of generalization and systematization of knowledge, skills and abilities. Forms of work: independent, frontal, group and individual. Methods: problem-search, verbal, visual, practical. Equipment: computer, screen, multimedia projector, self-control sheets, cards with tasks for each stage of the lesson. Appendix: lesson presentation. Lesson stages: 1. Organizational moment. 2. Determining the topic and purpose of the lesson. 3. Repetition and updating of basic knowledge. 4. Application of existing knowledge when solving problems. 5. Test work with mutual verification. 6. Homework assignment. 7. Reflection.
Lesson progress:
1. Organizational moment. (3 min) Teacher: Good morning, good people! May our lesson be fruitful! Smile and sit down! Guys, this year we started studying one of the oldest and most interesting sciences - geometry. In geometry lessons we became acquainted with a geometric figure, the name of which is encrypted in the rebus: Slide 2
Correct triangle. This is a very beautiful and interesting figure that holds many secrets, like the Bermuda Triangle, in which ships and planes still disappear and no one can explain the reasons for these phenomena. If you look closely and look at the world around you, you can find many outlines of this triangle. The triangle is one of the first geometric figures that began to be used in the ornaments of ancient peoples. Even one of the constellations in the sky has the shape of a triangle. Engineers love the triangle for its strength and use it to create structures, such as the Eiffel Tower and Ostankino TV Tower, various bridges and towers. The boom of a tower crane is secured with steel ropes, forming a triangle shape (as the story progresses, you can show slides 3, 4, 5). 2. Determination of the topic and purpose of the lesson. Here is a crossword puzzle, after solving which you will repeat the definitions and find out the topic of the lesson. (5 min) Slide 6.
1. The bisector segment of an angle connecting the vertex of a triangle with a point on the opposite side is called... 2. When proving the equality of two triangles, three... triangle equalities are used. 3. What is the name of the side in an isosceles triangle that is not equal to the other two? 4. A segment connecting the vertex of a triangle with the middle of the opposite side is called... a triangle 5. What is the name of a geometric figure consisting of three points connected by segments? 6. What is the name of the perpendicular drawn from the vertex of a triangle to the line containing the opposite side? 7. What is the name of a triangle in which all sides are equal? 8. Equal sides of an isosceles triangle are called... 9. What is the name of a triangle whose two sides are equal? Assessments on the self-control sheet On your desks there are assessment sheets, where you, as always, will give yourself grades during the lesson for each type of work. Give yourself a grade for the crossword puzzle. If there are no errors – “5”, 1.2 errors – “4”, 3.4 errors – “3”, 5 or more errors – “0”. Who has “5”, “4”? Hands up. What keyword did you come up with? (equality) Slide 7.
Using the keyword, and words numbered 2 and 5, formulate the topic of the lesson. The topic of our lesson is “Signs for the equality of triangles”. Slide 8. What goals will we set?
1. Repeat the signs of equality of triangles. 2. Strengthen the ability to solve problems using these characteristics. Write down the date, class work and topic of our lesson in your notebooks. 3.Repetition and updating of basic knowledge.
1.Front survey of students (3 min). Each of you has score sheets on your desk. We will work with them throughout the lesson. For each correct answer - 1 point. 1) Formulate the first sign of equality of triangles. 2) Formulate the second criterion for the equality of triangles. 3) Which triangle is called equilateral? 4) Which triangle is called isosceles? Name the elements of an isosceles triangle. 5) Formulate the third criterion for the equality of triangles. 6) What angles are called vertical? 7) Define adjacent angles. What properties does an isosceles triangle have? 9) Which segment is called the bisector of a triangle? 10) Which segment is called the median of a triangle? 11) Which segment is called the height of the triangle? 12) Define vertical angles. — Theoretically, you are well savvy. Let's see how attentive you are. We will play a “Yes-No” game. I will hand out task cards. 2. Performing the game “Yes - No” (4 min) If the statement is correct, write “yes”, if a mistake is made, then no. Remember that in definitions and theorems there are words without which the definitions and theorems do not lose meaning, but there is a word without which the sentence does not carry any information. You are given 3 minutes to complete the task. Get started. Option 1. 1. Is it true that if the triangles are equal, then each angle of the first triangle is equal to each angle of the second triangle? (no) 2. Is it true that for each side of the first triangle you can find a side equal to it in the second, equal triangle? (yes) 3. Is it true that if the side and angle of one triangle are respectively equal to the side and angle of another triangle, then such triangles are congruent? (no) 4. Is it true that if three sides of one triangle are respectively equal to three sides of another triangle, then such triangles are congruent? (yes) 5. Is it true that if two angles of one triangle are respectively equal to two angles of another triangle, then such triangles are congruent? (no) Option 2. 1. Is it true that if the triangles are equal, then each side of the first triangle is equal to each side of the second triangle? (no) 2. Is it true that for each angle of the first triangle you can find an angle equal to it in the second, equal triangle? (yes) 3. Is it true that if the side and two angles of one triangle are respectively equal to the side and two angles of another triangle, then such triangles are congruent? (no) 4. Is it true that if three angles of one triangle are respectively equal to three angles of another triangle, then such triangles are congruent? (no) 5. Is it true that if two sides and the angle between them of one triangle are respectively equal to two sides and the angle between them of another triangle, then such triangles are congruent? (yes) - Exchange cards with your desk neighbor, check the answers, give marks with a pencil, comment on the results, correct mistakes. (Work in pairs, frontal work). The correct answers for each option are displayed on the slide. Slide 9. The testing student gives a grade according to the following criteria: 5 correct – “5”, 4 correct – “4”, 3 correct – “3”, 2 or 1 correct – “2”. Put the received mark on the evaluation sheet. — Raise your hands, those who received - 5, those who received - 4, those who received - 3. We continue to work. — Guys, for the first year you have been studying the subject of geometry and you realized that the subject of studying this section of mathematics is solving problems based on the studied definitions, properties and theorems. Slide 10. 4. Application of existing knowledge when solving problems. The famous teacher and mathematician György Pólya said: “If you want to learn to swim, then boldly enter the water, and if you want to learn to solve problems, then solve them.” (D. Polya) How do you understand these words? Task 1. Working from ready-made drawings “Continue the solution” (5 min) A drawing is depicted on the board for each row, the beginning of the solution to the problem is given, and the students of each row need to complete the solution to this problem. Students think for 1-2 minutes, deliberate in pairs, and then one representative from each row comes out and explains the solution to the problem. Slide 11.
We will work with them throughout the lesson. For each correct answer - 1 point. 1) Formulate the first sign of equality of triangles. 2) Formulate the second criterion for the equality of triangles. 3) Which triangle is called equilateral? 4) Which triangle is called isosceles? Name the elements of an isosceles triangle. 5) Formulate the third criterion for the equality of triangles. 6) What angles are called vertical? 7) Define adjacent angles. What properties does an isosceles triangle have? 9) Which segment is called the bisector of a triangle? 10) Which segment is called the median of a triangle? 11) Which segment is called the height of the triangle? 12) Define vertical angles. — Theoretically, you are well savvy. Let's see how attentive you are. We will play a “Yes-No” game. I will hand out task cards. 2. Performing the game “Yes - No” (4 min) If the statement is correct, write “yes”, if a mistake is made, then no. Remember that in definitions and theorems there are words without which the definitions and theorems do not lose meaning, but there is a word without which the sentence does not carry any information. You are given 3 minutes to complete the task. Get started. Option 1. 1. Is it true that if the triangles are equal, then each angle of the first triangle is equal to each angle of the second triangle? (no) 2. Is it true that for each side of the first triangle you can find a side equal to it in the second, equal triangle? (yes) 3. Is it true that if the side and angle of one triangle are respectively equal to the side and angle of another triangle, then such triangles are congruent? (no) 4. Is it true that if three sides of one triangle are respectively equal to three sides of another triangle, then such triangles are congruent? (yes) 5. Is it true that if two angles of one triangle are respectively equal to two angles of another triangle, then such triangles are congruent? (no) Option 2. 1. Is it true that if the triangles are equal, then each side of the first triangle is equal to each side of the second triangle? (no) 2. Is it true that for each angle of the first triangle you can find an angle equal to it in the second, equal triangle? (yes) 3. Is it true that if the side and two angles of one triangle are respectively equal to the side and two angles of another triangle, then such triangles are congruent? (no) 4. Is it true that if three angles of one triangle are respectively equal to three angles of another triangle, then such triangles are congruent? (no) 5. Is it true that if two sides and the angle between them of one triangle are respectively equal to two sides and the angle between them of another triangle, then such triangles are congruent? (yes) - Exchange cards with your desk neighbor, check the answers, give marks with a pencil, comment on the results, correct mistakes. (Work in pairs, frontal work). The correct answers for each option are displayed on the slide. Slide 9. The testing student gives a grade according to the following criteria: 5 correct – “5”, 4 correct – “4”, 3 correct – “3”, 2 or 1 correct – “2”. Put the received mark on the evaluation sheet. — Raise your hands, those who received - 5, those who received - 4, those who received - 3. We continue to work. — Guys, for the first year you have been studying the subject of geometry and you realized that the subject of studying this section of mathematics is solving problems based on the studied definitions, properties and theorems. Slide 10. 4. Application of existing knowledge when solving problems. The famous teacher and mathematician György Pólya said: “If you want to learn to swim, then boldly enter the water, and if you want to learn to solve problems, then solve them.” (D. Polya) How do you understand these words? Task 1. Working from ready-made drawings “Continue the solution” (5 min) A drawing is depicted on the board for each row, the beginning of the solution to the problem is given, and the students of each row need to complete the solution to this problem. Students think for 1-2 minutes, deliberate in pairs, and then one representative from each row comes out and explains the solution to the problem. Slide 11.
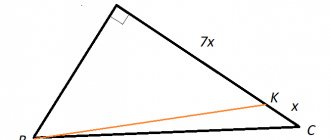
Task 2. Oral solution of problems using ready-made drawings (6 min) We repeated how to solve problems. We solve problems orally using ready-made drawings. . ” Who is first? Slide 12 1. 2. Listen carefully as you evaluate each other. Task 3. Solving problems with complete solutions. (6 min): - In geometry, it is very important to be able to look and see, notice and note various features of geometric figures. One student solves the problem on the board, and the rest in notebooks. Slide 13 Task 1.
Task 2. Slide 14.
4. Physical education session to the music “Yellow leaves are circling over the city” (2 min) Slide 15. Inhale and exhale, stretch. Hands up, work with your fingers to form various triangles. Draw a triangle in the air with your left hand, then with your right, and then with both. Paired with a neighbor (I have two hands - two sides, the neighbor helps to make a triangle). Draw a triangle on the floor with each leg. Shake off the fatigue from your arms and legs. We sat down. 5. Test work (6 min.) Slide 16-17. For each correct answer, the participant receives 1 point. I wish you success. 1. Indicate which of the following pictures have equal triangles, by what criteria are they equal? 2. By what criteria are triangles congruent? a) on two sides and the angle between them b) on a side and two adjacent angles c) on three sides
Answers: Slide 18. 1. I, III - along two sides and the angle between them III, IV - along a side and two adjacent angles 2. According to I, the triangles in Fig. are equal. 1,4,5,8. According to the II criterion, the triangles in Fig. 3,6,9 are equal. according to the III criterion, triangles are equal 2.7 6. Homework: (1 min) (cards). Slide 19 Solve problems using ready-made drawings 1.
2.
8 Reflection. (3 min) Slide 20 Give yourself a final grade for your work in the lesson as a whole.
What did you get? Very good! What goals did we set for ourselves? Have we achieved them? Grading. You all have triangles on your tables. Those who liked our lesson and who think that they did a good job, show the green triangle; those who didn’t quite like our lesson or were less fortunate than others, show the yellow triangle. Download lesson notes on geometry in 7th grade. “Triangles. Signs of equality of triangles"
Presentation on the topic: Triangles. Signs of equality of triangles
We recommend watching:
Notes for a 7th grade geometry lesson on the topic “Relationships between the sides and angles of a triangle.” Notes for a 7th grade geometry lesson with presentation. Triangles Lesson notes for an integrated lesson in geometry and the Russian language with presentation, grade 7. Geometry lesson notes, grade 7. The third sign of equality of triangles
Similar articles:
Geometry lesson notes for 7th grade
Math quizzes with answers, 7th grade
Lesson summary “The third sign of equality of triangles”
teacher:
Maksudova Indira Ryzabekovna
item:
geometry
Class:
7th grade
Topic: "The third sign of equality of triangles"
lesson type:
discovery of new knowledge.
target:
acquaintance with the third sign of equality of triangles.
Tasks
subject:
study the third criterion for the equality of triangles, teach students to apply the third criterion for the equality of triangles when solving problems, and, with the help of practical knowledge, ensure that students understand the differences between the first, second and third signs for the equality of triangles.
activity:
develop students’ skills in proving statements using practical actions and previously learned concepts and theorems;
to develop in students the ability to apply signs of equality when determining the equality of triangles;
developing:
to form key competencies of students: information (the ability to analyze information, compare, draw conclusions), problem-solving (the ability to pose problems and, using existing knowledge, find a way out of the situation); communicative (ability to work in groups, ability to listen and hear others, accept the opinions of others)
Equipment:
multimedia projector, handouts, triangle templates, class cards, clusters
Organizing time.
Hello! Today there are guests at our lesson, turn to face them, silently greet them, smile, sit down. (Slide No. 1)
Motivation.
I want to start the lesson with a question: Does anyone know why they say that a triangle is a rigid figure? No? I think we'll get the answer at the end of the lesson.
Updating knowledge. —
So, let's start with the first point. In order to begin to learn something new, you need to answer the question “What do I know?”
Exercise 1.
(Appendix 2) Everyone has a printout on their desk. We read, immediately inserting the necessary words. (Slide No. 2)
Fill in the missing word.
- In geometry, shapes are called _____________ if they coincide when superimposed.
- A figure consisting of three points not lying on the same straight line, segments connecting these points, is called ________________________
- Triangle elements: ______________, _______________, ___________
- In triangle ABC, the angle between sides AB and AC is:________
- The angles adjacent to side BC of triangle ABC are:_____, ______
- A statement whose truth requires proof is called __________________ (slide No. 3)
Task2.
Oral exercises:
1) is the statement true:
- “If the triangles are equal, then are their perimeters equal?”
- “The perimeters of the two triangles are equal. Are these triangles equal? "
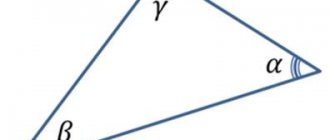
2) In the figure, find the corresponding equal sides and angles and name them. How many equalities did we get? (slide No. 4)
Statement of a problem situation.
Goal: increasing learning motivation, posing a problem situation.
Now look carefully at the drawing. (slide No. 5) Find triangles that are equal according to the first or second criterion for the equality of triangles. Formulate these signs. Are there any other equal triangles?
And do you think this is another sign?
Then try to formulate the topic of the lesson. (slide number 6) Let's write down the date and topic of the lesson in our notebooks.
What is the goal of our lesson? (introduction to the third sign)
What tasks should we set? (repeat signs 1 and 2, prove sign 3, learn to apply it when solving problems) (slide No. 7)
Discovery of new knowledge.
(I'll find out on my own!)
Goal: leading students to independently formulate the signs of equality of triangles and the method of proof.
Problematic question:
Is it enough to have three pairs of equal sides in order to conclude that these triangles are equal?
Once again, which triangles are called congruent? Let's try to prove or disprove our assumption using a practical method. You have cut out triangles with equal sides on your tables, try to combine them. Are they equal?
Who will try to formulate the third criterion for the equality of triangles?
Let's open the textbooks p.72. Let's read the third sign.
What should we do? (prove).
The proof on the clusters is pre-printed, shuffled and on the side of the board with magnets. We will collect the children in the correct order and arrange them first on the board, then in notebooks.
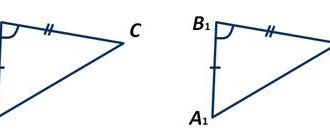
Drawing on the board itself (in advance) Given: ABC; A1B1C1, AB=A1B1, BC=B1C1, AC=A1C1
Prove: ABC=A1B1C1
Proof:
Let us arrange the triangles ABC and A1B1C1 so that vertex A coincides with vertex A1, vertex B coincides with B1, and vertices C and C1 lie in different half-planes relative to straight line AB. Let's draw segment CC1. AC = A1C1, triangle C1A1C is isosceles, which means angle1 = angle2. Similarly, angle 3 = angle 4. This means angle A1C1B1 = angle A1CB1. According to the first sign of equality of triangles, triangle A1C1B1 = A1CB1
Once again formulate the 3rd criterion for the equality of triangles.
Physical education minute
for the eyes: (music)
Close your eyes for a few seconds, strongly tensing your eye muscles, then open them, relaxing the muscles. Repeat 3-4 times. look at the bridge of your nose and hold your gaze. Then look into the distance. Repeat 3-4 times. Slowly tilt your head: forward-left-right-back. repeat 3-4 times. Blink your eyes several times without straining your muscles. take deep breaths and exhale slowly.
Primary application of the acquired knowledge. (I’ll try to apply it!)
Goal: clarifying the algorithm for using new knowledge and incorporating it into the student’s knowledge system. Solving problems using ready-made drawings. It is necessary to determine the equality of these triangles.
Exercise 1.
(The board is divided into three parts: I, II, III sign of equality of triangles)
Let's continue talking about the signs. We looked around, there were cards with pictures hanging on the walls in the office, it was necessary to determine the sign of equality of triangles and place them on the board accordingly. There are enough cards for everyone. For the first group, cards with blue numbers, for the second group with green numbers, for the third group with red numbers.
Task2.
Cards are distributed among the groups to prove the third criterion. Discussion 1 minute. One of the group proves verbally.
Consolidation: “I can handle it!”
(Independent work, after which students carry out mutual checking using a finished sample)
| Prove that the triangles are congruent, fill in the blanks in the notes. | ||
| ΔPRS = ΔKMN, on __ basis because: | ΔADB = ΔBDC, On __ basis because | ΔВOD= ΔAOC, By__ basis. because |
| 1. = | 1. = | 1. = |
| 2. L = | 2. = | 2. = |
| 3. L = | 3. – general | 3. L = |
Sample answer: (slide No. 8)
| Prove that the triangles are congruent, fill in the blanks in the notes. | ||
| ΔNNP = ΔPRQ, according to II sign because: | ΔADB = ΔBDC, according to III sign because | ΔАВС= ΔDBC, by I sign . because |
| 1. RS = KN | 1. AD = DC | 1.BO = OC |
| 2. L S | 2. AB = BC | 2. DO = OA |
| 3. L R = | 3. DB – general | 3. L BOD= |
Application of the third sign of equality of triangles in everyday life and in production. Emil Tazhiev prepared for us a presentation “Triangle is a rigid figure”
Reflection: (slide number 9)
- I learned (learned)…
- The most interesting part of the lesson for me was...
- I would like to know more...
Grading (slide No. 10)
Number of total points:
from 21 to 23 points – score “5”
from 18 to 20 points – score “4”
If the number of points is below 18 points, then we will continue working on mastering this material in subsequent lessons.
Homework: (slide No. 11) p. 11 learn the theorem, No. 255, 257, 260.
Slide No. 12 Thanks for the lesson!